The beginning of an indepth study of graphing quadratic equations (parabolas) Includes the vocab words vertex and axis of symmetry Graph of the parabola in vertex form The vertex form of parabola equation is y = a(x h)^2 k , where ( h , k ) = vertex and axis of symmetry x = h The parabola is f(x) = y = 2x 2Algebra Quadratic Equations and Functions Quadratic Functions and Their Graphs 1 Answer Nallasivam V Refer explanation section Explanation Given #y=2x^24# To find the vertex, rewrite the function as
Parabolas Xcelerate Math
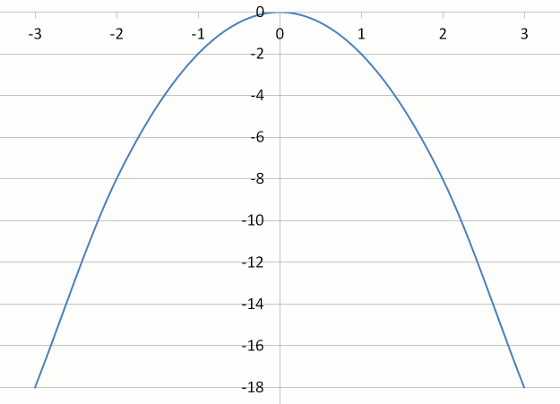
Graph the parabola y=2x^2-5x-3
Graph the parabola y=2x^2-5x-3-Also graph the parabola If you can please include the points that I have to graph/plot that would be great I know how to get the vertex but I always mess up when it comes to graphing the exact point or points Answer by ewatrrr() (Show Source)The focus of a parabola can be found by adding to the ycoordinate if the parabola opens up or down Substitute the known values of , , and into the formula and simplify Find the axis of symmetry by finding the line that passes through the vertex and the focus
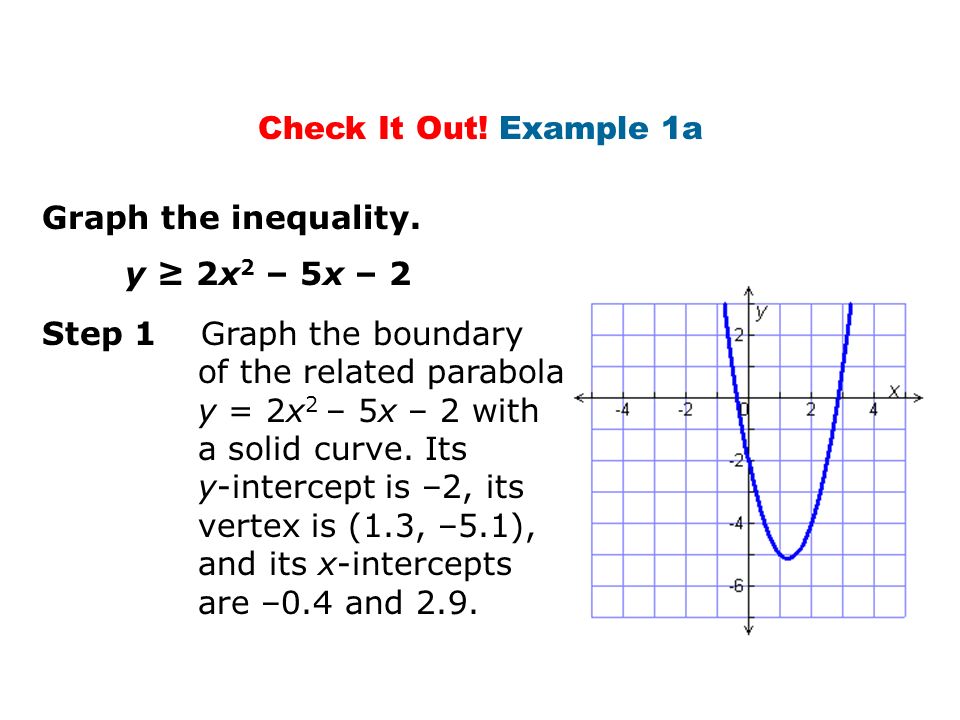



Warm Up 1 Graph The Inequality Y 2x 1 Solve Using Any Method 2 X 2 16x 63 X 2 8x 3 7 Ppt Download
Graph y=2x^216x28 y = 2x2 − 16x 28 y = 2 x 2 16 x 28 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for 2 x 2 − 16 x 28 2 x 2 16 x 28 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find the values of a aJenna from SVSU Micro Math helps you graph a parabolaProblem Graph the parabola y = 2x^2 2x 3Level intermediate/college algebra#SVSUmicromathLet's graph y=2x 2x (red) and y=2x 24x (blue) The vertex when a =2 and b =1 is at x=¼, and the vertex when a =2 and b =4 is x=1 Now, we see that our hypothesis is not completely correct, for – b /2 for the first graph would give us ½, but we have ¼ Instead, it must be – b /2 a since a =1 on our previous graphs and that works
Algebra > Graphs> SOLUTION Graph the parabola y=2x^24x6 on graph paper Log On Algebra Graphs, graphing equations and inequalities Section Solvers SolversGraph the parabola and give its vertex, axis of symmetry, intercepts, and y intercept y = 3x^2 6x 10 The vertex is (Type an ordered pair) The axis of symmetry is Type an equation Use integers or Select the correct choice below and fill in any answer boxes within your choice The xintercepts are at x (Type an exact answer, using radicalsClick here to see ALL problems on Graphs Question 6355 Graph the parabola y= 3/2 x^2 Answer by MathLover1 () ( Show Source ) You can put this solution on YOUR website!
View interactive graph > Examples (y2)=3(x5)^2;To find the vertex, we first need to find the axis of symmetry (ie the xcoordinate of the vertex) To find the axis of symmetry, use this formula From the equation we can see that a=2 and b=8 Plug in b=8 and a=2 Multiply 2 and 2 to get 4 Reduce So the axis ofQuestion Graph the parabola y=x^210x Plot the vertex and four additional points, two on each side of the vertex Answer by josgarithmetic() (Show Source) You can put this solution on YOUR website!



Solution Sketch The Graph Of The Function Y 2x 2 4x 5



Key To Practice Exam Ii
Graph the parabola y = 2x^2 To graph the parabola, plot the vertex and four additional points, two on each side of the vertex Then click on the graph icon The standard form of parabola equation is y = a(x h)^2 k, where (h, k) = vertex and axis of symmetry x = h The parabola is y = x 2 6x Wrote the equation in standard form of a parabola eqaution To change the expression x 2 6x into a perfect square trinomial add and subtract (half the x coefficient)² Here x coefficient = 6 so, (half the x coefficient)² = (6/2) 2 = 9Below is an animation of the lines tangent to y=x^2 at each point



Quadratics In Context Nz Maths




What Is The Graph Of The Parabola Represented By Y 2x 2 8x 9 Socratic
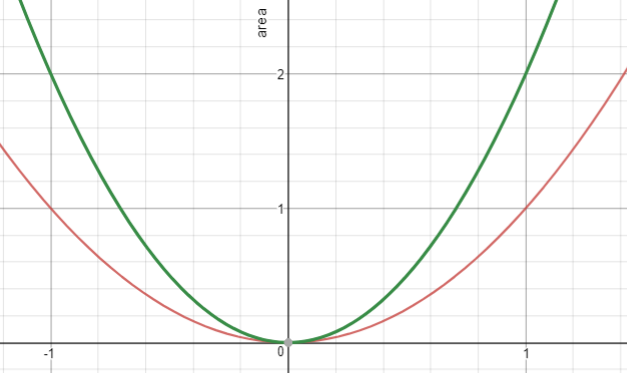
Identify at least the xcoordinate of the vertex How do you graph the parabola #y= 2x^24# using vertex, intercepts and additional points?The graph of a quadratic equation in two variables (y = ax 2 bx c) is called a parabolaThe following graphs are two typical parabolas their xintercepts are marked by red dots, their yintercepts are marked by a pink dot, and the vertex of each parabola is marked by a green dot



Solution Graph The Quadratic Equation Y 2x 2 What Is The Vertex Also Graph The Parabola If You Can Please Include The Points That I Have To Graph Plot That Would Be G




Vertex Axis Of Symmetry Of A Parabola Video Khan Academy
Released under CC BYNCSA http//creativecommonsorg/licenses/byncsa/30/legalcodeGraphing a basic parabola using y=3x^2 to show the use of a table and keGraph y^2=x y2 = x y 2 = x Rewrite the equation as x = y2 x = y 2 x = y2 x = y 2 Divide each term by and simplify Tap for more steps Divide each term in x = y 2 x = y 2 by x = y 2 x = y 2 Cancel the common factor ofGraph the parabola and give its vertex, axis, xintercepts, and yintercept y = 2x^2 32x 126 The vertex is x= 0 x = 2 x = 7 x = 8 Select the correct below and fill in any answer boxes within your choice The xintercepts are at x = There is no xintercept The yintercept is at y = Chose the correct graph of the function y = 2x^2 32x 126 below



Solution Graph Y 2x 2 4x



Quadratics Graphing Parabolas Sparknotes
The equation of parabola can be expressed in two different ways, such as the standard form and the vertex form The standard form of parabola equation is expressed as follows f (x) = y= ax2 bx c The orientation of the parabola graph is determined using the "a" value If the value of a is greater than 0 (a>0), then the parabola graphIn the figure, the vertex of the graph of y=x 2 is (0,0) and the line of symmetry is x = 0 Definition Parabola 1Algebric A Parabola is the graph of a quadratic relation of either form where a ≠ 0;Question 6525 Graph the quadratic equation y=2x^2 What is the vertex?



What S The Axis Of Symetry Vertex And Graph Y 2x 2 6x 1 Mathskey Com




Please Help Thank You The Graph Of The Function Y X2 Is Shown How Will The Graph Change If Brainly Com



0 件のコメント:
コメントを投稿